Crystallographic restriction
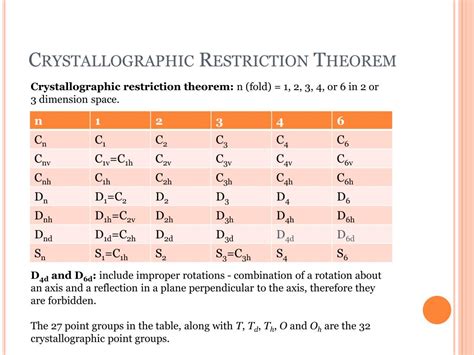
The general crystallographic restriction on rotations does not guarantee that a rotation will be compatible with a specific lattice. For example, a 60° rotation will not work with a square lattice; nor will a 90° rotation work with a rectangular lattice. See more The crystallographic restriction theorem in its basic form was based on the observation that the rotational symmetries of a crystal are usually limited to 2-fold, 3-fold, 4-fold, and 6-fold. However, quasicrystals can … See more The special cases of 2D (wallpaper groups) and 3D (space groups) are most heavily used in applications, and they can be treated together. Lattice proof A rotation symmetry in dimension 2 or 3 must move a lattice … See more • Crystallographic point group • Crystallography See more • The crystallographic restriction See more When the dimension of the lattice rises to four or more, rotations need no longer be planar; the 2D proof is inadequate. However, … See more The crystallographic restriction theorem can be formulated in terms of isometries of Euclidean space. A set of isometries can form a See more 1. ^ Shechtman et al (1982) See more WebApr 25, 2024 · So, in many cases, the residency and employment restrictions are worse than the registry itself. Most of these restrictions contain 1,000-foot rules. A thousand …
Crystallographic restriction
Did you know?
WebSep 20, 2024 · The crystallographic restriction theorem in its basic form was based on the observation that the rotational symmetries of a crystal are usually limited to 2-fold, 3-fold, 4-fold, and 6-fold. However, quasicrystals can occur with other diffraction pattern symmetries, such as 5-fold; these were not discovered until 1982 by Dan Shechtman. [1] … WebDec 13, 2024 · Crystallographic restriction theorem The crystallographic restriction theorem generally states that the rotational symmetries of a crystal are limited. We have …
WebThe crystallographic restriction We now look at which groups can be the symmetry groups of lattices. Note that if L is the lattice of translations of a symmetry group G then … WebAug 15, 2024 · Crystallography Restriction theorem Asked 3 years, 6 months ago Modified 3 years, 6 months ago Viewed 303 times 1 I'm trying to understand the Crystallography Restriction theorem, but most proofs I have found include some assumptions that are non-obvious to me.
WebNov 9, 2015 · The only theorem in our notes about Crystallographic restriction is: Theorem: (Crystallographic restriction) If L ⊂ R 2 is non-trivial and H ⊂ O 2 is a group … WebApr 14, 2024 · The entropic gain that results from the conformational restriction could account for the faster association rate of c-Epi compared to Epi with the β 2 AR. c-NorEpi also displays a modest ~2-fold ...
WebFeb 1, 2002 · In its simplest incarnation, the crystallographic restriction says that if A is a 2 × 2 matrix with integer coefficients and A n = id for some natural number n, then n = 1, 2, 3, 4 or 6. For more...
http://snst-hu.lzu.edu.cn/zhangyi/ndata/Crystallographic_restriction_theorem.html#:~:text=The%20crystallographic%20restriction%20theoremin%20its%20basic%20form%20is,a%20list%20of%20independent%20finite%20translations%20%28Coxeter%202489%29. diatomaceous earth in tamilWebThe Crystallographic Restriction, Permutations, and Goldbach’s Conjecture John Bamberg, Grant Cairns, and Devin Kilminster 1. INTRODUCTION. The object of this paper is to make an observation connecting Goldbach’s conjecture, the crystallographic restriction, and the orders of the elements of the symmetric group. diatomaceous earth is formed byWebCrystallographic restriction theorem This article is missing information about a succinct statement of the theorem. Please expand the article to include this information. Further details may exist on the talk page. (December 2024) The crystallographic restriction theorem in its basic form was based on the observation that the rotational ... citing cdc apa stylehttp://www-groups.mcs.st-andrews.ac.uk/~john/geometry/Lectures/A2.html diatomaceous earth kenyaWebJan 28, 2014 · The crystallographic restriction theorem was based on the observation that the rotational symmetries of a crystal are usually limited to 2-fold, 3-fold, 4-fold, and 6-fold. One way to prove this … diatomaceous earth in sand filterWebOct 24, 2024 · The general crystallographic restriction on rotations does not guarantee that a rotation will be compatible with a specific lattice. For example, a 60° rotation will not … citing ccrWebDec 13, 2024 · The crystallographic restriction theorem generally states that the rotational symmetries of a crystal are limited. We have seen from a previous section that a lattice is formed by repeating lattice points that have the same environment. citing cdc page